(This contains a repository of pdf files for my personal use. Please note that you are legally permitted to download the papers only if you or your institution are subscribed to the respective journals.)

Control theory is a field of great practical importance with well-established theoretical fundations for systems described by ordinary differential equations (ODEs). However, many systems cannot be adequately modelled by using ODEs and one needs to use instead partial differential equations (PDEs). Systems that are described by PDEs include fluid mechanics model, channel flows, convection problems, magnetohydrodynamic problems, etc... These systems belong to the class of Distributed Parameter Systems (i.e., systems whose state is infinite-dimensional, also including delay systems). For distributed parameter systems, control theory is still under development and there are many challenges (both theoretical and practical) that need to be tackled.
Backstepping is a powerful tool coming from nonlinear control theory that is proving to be of great utility for designing boundary controllers and observers that solve some of these open problems, and remarkably so for many types of evolution equations (both parabolic and hyperbolic). Recent extensions of the method include coupled systems, two or more dimensions, and/or to complicated geometries.
Some selected publications:
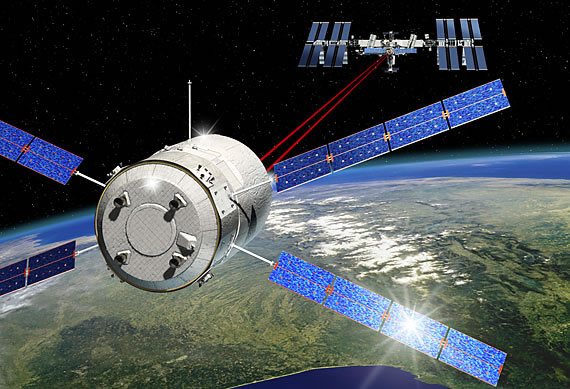
On-orbit spacecraft rendezvous, this is, the controlled encounter of two or more spacecraft in space, is an extremely important practical problem that has already been put to practice in numerous space missions. For instance the Apollo program required the rendezvous between the command module and the lunar module, and the development of techniques for solving the rendezvous problem was an important milestone for the American space program.
Today, the main challenge of spacecraft rendezvous consists on developing an autonomous system with low weight and consumption able to obtain a safe encounter without wasting any fuel. Numerous optimization and control techniques can be applied to achieve a successful rendezvous. However modern automatic rendezvous systems are rather heavy and expensive and it is still a challenge to develop light, low-cost systems for small satellites that would allow them, for instance, to rendezvous with large spacecraft such as space stations.
Advanced topics include six-degree-of-freedom rendezvous (this is, rendezvous with controlled attitude), on-off thrusters, robustness, event-based control, or rendezvous in the three body circular restricted problem.
Some selected publications:

The age of Unmmaned Aerial Vehicles (UAVs), popularly known as drones, is already here. With it, a huge number of applications are arising both in the civil and military fields. UAVs require well-designed guidance, control and navigation (GNC) systems, able to carry out successfully any mission within the vehicle capabilities.
In particular, flight controllers are of paramount importance to maintain a stable flight with good levels of performance. While there are already commercial generic systems with decent performance that can be installed on board to carry out the GNC functions, they are usually rather expensive and oftentimes require extensive knowledge of aircraft aerodynamics, which requires wind tunnel experiments or even flight testing. However, nowadays it is possible (even with off-the-shelf hardware and at a low cost) to develop algorithms that do not require any a priori knowledge of aircraft aerodynamics and are even able to cope with sudden system changes due to faults or reconfiguration. One example of such algorithms is adapative control, in particular adaptive backstepping.
Guidance algorithms (the high level systems that issue references for the flight controllers) pose a different set of challenges. For instance, a shortcoming of typical commercial systems is that they tend to degrade considerably in the presence of wind and/or uncertainties, or are unable to provide time synchronization capabilities. It is however possible to develop predictive guidance algorithms with more functionalities than conventional systems, that are able to overcome uncertainties and include time synchronization in a cost-optimal way, using for instance Model Predictive Control techniques.
Some selected publications:
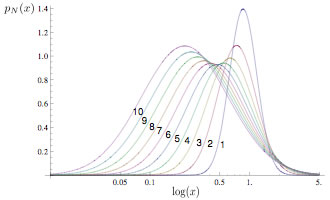
Real systems are characterized, among other things, by the ubiquitous presence of uncertainty. Be it in the form of measurement errors, unmodelled dynamics, unknown system parameter, or errors in the initial conditions (or several of these at the same time), in applications it is just unavoidable to have a certain degree of uncertainty present in the system.
However, oftentimes unknown parts of the systems or parameters can be modelled by making use of the theory of statistics, in particular by using distribution functions. The inclusion of probabilistic modelling makes the plant dynamics stochatic (be it continuous or discrete in time).
There is a theoretical body that allows, from these stochastic models, to determine the system evolution. The state of the system would, however, be known not in an exact way, but rather described in a probabilistic way (as a distribution function that evolves in time). This theory of stochastic differential equations also allows us to quantify uncertainty propagation, i.e., how uncertainty rises (or diminishes) with time.
In some problems, filtering can be used to reduce uncertainty with the use of measurements.
Applications are very diverse, ranging from evolution of droplet populations to the dynamics of aircraft when subject to uncertainty in the performance models or the initial conditions, ATM, or orbits around asteroids with uncertain gravity field.
Some selected publications:

The number of Earth Observation Satellites (EOSs) is on the rise, mainly due to the ever-increasing demand of images of our planet. EOSs are required to capture these pictures with as much resolution as possible and, oftentimes, as soon as possible or even on the fly (for example when catastrophic events happen).
Managing these observational resources has been done in the past mostly in a manual fashion, and on a satellite-by-satellite basis. However, there is nowadays a true market for satellite images that allows the combination of observations from many different satellites to cover any region of interest. Selecting a set of satellites in an optimal cost-effective way, taking into account observational constraints, and in a short time-frame, require the development of optimal planners able to tackle challenging computational geometry problems together with orbital mechanics and the user preferences.
There are other instances of optimal planning problems in space missions such as the satellite-antenna assignation problem (for a large number of both antennas and satellites) or the management of observational modes for agile satellites, which requires the coordination of the satellite attitude with the relative position of the different targets as the satellite moves along its orbit.
All these applications require the development of heuristic algorithms which are able to rapidly provide feasible solutions (approaching as much as possible the global optimum). These algorithms pose a challenge as they have to be integrated with tools from computational geometry and orbital mechanics and verified and simplemented in realistic simulation software (for instance, SaVoir).
Some selected publications:
© 2007-2023 Rafael Vazquez Valenzuela